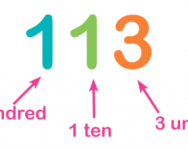
What is partitioning?

Partitioning is a way of working out maths problems that involve large numbers by splitting them into smaller units so they’re easier to work with. So, instead of adding numbers in a column, like this…

  79
+34
113

…younger students will first be taught to separate each of these numbers into units, like this…

70 + 9 + 30 + 4

…and they can add these smaller parts together. For instance, they can pick out all the tens and work down to single units, making the problem more and more manageable, like this…

70 + 30 + 9 + 4 = 100 + 13 = 113

Children are taught this method before they learn to add numbers in columns. Partitioning gives children a different way of visualising maths problems, and helps them work out large sums in their head. By breaking numbers down into units that are easy for them (and us!) to calculate mentally, they can reach the correct answer without counting out tricky double or triple-digit numbers on their fingers or trying to remember where a decimal point needs to be.

Partitioning is taught in Key Stage 1, to make children aware that a two-digit number is made up of tens and ones. Teachers often use arrow cards for this so that children can physically make a number, such as 24, out of a 20 and a 4.  The idea is that the child lines up the arrows together to make the numbers fit:

These are two commonly used methods for adding larger numbers:

 
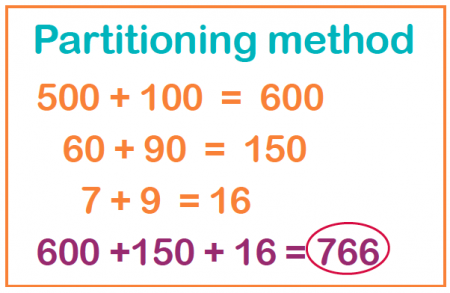
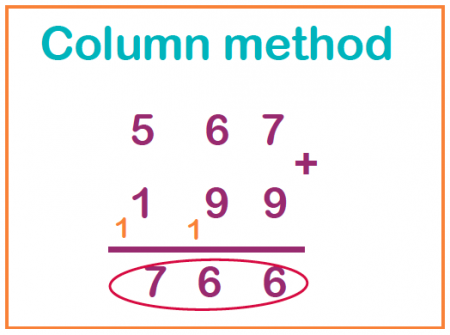
A teacher might start teaching children to add two-digit and three-digit numbers in Year 3 by partitioning. The reason for this is that it helps children to mentally add multiples of ten (70 + 50 for example) and multiples of 100 (400 + 800 for example). Children in Year 3 should add also learn to add three-digit numbers using the column method, so your child is likely to encounter both of these methods.

Partitioning in multiplication

Children in Year 3 will also need to multiply two-digit numbers by a one-digit number. They will usually be taught this by partitioning, for example:

37 x 4 =
30 x 4 = 120
7 x 4 = 28
120 + 28 = 148

As children move into Year 4 and 5, they have to start multiplying two two-digit numbers. There are two commonly used methods for this (the grid and column methods); the grid method uses partitioning:

Again, the grid method is used so that children are repeatedly practising multiplying multiples of ten with other numbers, for example: 30 x 20, 30 x 3, 20 x 8, etc. Once teachers are very confident that a child is aware of how to multiply multiples of ten and one hundred, they will often allow a child to move onto the quicker column method.

In Year 6, children need to start calculating with decimals. To make this easier, a teacher may show them how to partition decimals. For example:
3.5 x 7
3 x 7 = 21
0.5 x 7 = 3.5
21 + 3.5 = 24.5