TheSchoolRun.com closure date
As we informed you a few months ago, TheSchoolRun has had to make the difficult decision to close due to financial pressures and the company has now ceased trading. We had hoped to keep our content available through a partnership with another educational provider, but this provider has since withdrawn from the agreement.
As a result, we now have to permanently close TheSchoolRun.com. However, to give subscribers time to download any content they’d like to keep, we will keep the website open until 31st July 2025. After this date, the site will be taken down and there will be no further access to any resources. We strongly encourage you to download and save any resources you think you may want to use in the future.
In particular, we suggest downloading:
- Learning packs
- All the worksheets from the 11+ programme, if you are following this with your child
- Complete Learning Journey programmes (the packs below include all 40 worksheets for each programme)
You should already have received 16 primary school eBooks (worth £108.84) to download and keep. If you haven’t received these, please contact us at [email protected] before 31st July 2025, and we will send them to you.
We are very sorry that there is no way to continue offering access to resources and sincerely apologise for the inconvenience caused.
SATs advice: maths problem-solving

Whatever the key stage, practice makes perfect when it comes to maths, so help your child to develop their skills using past exam papers (and use our tips to help you get the best out of using KS2 maths SATs past papers at home).
Problem-solving in Key Stage 1 maths


Prepare your child for SATs today
- Your guide to SATs
- KS1 & KS2 SATs revision courses
- SATs practice papers in English & maths
For example, children might come across a problem like this one:
Jane wants to buy a pack of biscuits costing 80p. She has 50p. How much more money does she need to buy the biscuits?
They might choose to solve this by working out 80 - 50, in which case, they might want to draw 8 circles, each representing 10, and then cross off 5 of them:
Alternatively, they might want to draw a number line and write 50p on the left hand side, then jump in tens until they get to 80p.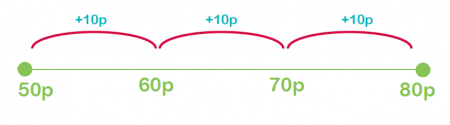
When practising word problems it is really important to allow your child to draw diagrams and pictures as much as possible. You may need to show them your own pictures to help them, but make it very clear that they don't have to do these problems in their head (unless they want to!).
Children will also come across problems involving multiplication and division. By the time they sit their KS1 SATs, they should know their 2, 5 and 10 times tables off by heart (as well as all the corresponding division facts that go with them). Although the word problems they come across in the SATs will test them on these facts, your child may not immediately realise that this is what is being asked of them. This is why it is again really important that they use diagrams to help themselves get their answer. For example:
I have three boxes. Each box contains five sweets. How many sweets do I have altogether?
Some children will know immediately that the question is asking 3 x 5, the answer to which is 15. Others may not see this immediately and will need to draw the three boxes and then perhaps write '5' on each one, then count in 5s to find the answer.
I need to buy 15 plastic cups for a party. A shop sells cups in packs of 6. How many packs will I need to buy?
This question is harder, as it is not immediately clear whether it is a multiplication or division question and it could be confusing to a child, as 15 cannot be divided by 6. Again, drawing a picture will help. Your child could draw a circle with a '6' in it, representing a pack of 6 cups. They could then draw another and add the two 6s together to make 12. At this point, they would realise that they need one more pack, which would make 18. This means that they would have enough cups to supply the 15 needed for the party.
Problem-solving in Key Stage 2 maths
Again, they will be given problems to solve involving all four operations. Some of the problems will involve money or measures. They will also need to know how to calculate with fractions, decimals and percentages. At this stage, it is fine for children to draw pictures and diagrams if this will help them, but they are expected to have efficient written methods for each operation. Many of the problems will have two or more 'steps' which means children will need to carry out two or more operations in order to work out the answer.
An example of a two-step problem they might have to do is as follows:
Year 5 has two classes: Class A and Class B. There are 26 children in Class A and 32 children in Class B. Exactly half of the children in Year 5 have packed lunches. How many children have packed lunches?
- In this case, they would need to realise that the first operation they would need to do is 26 + 32 in order to find out how many children were in Year 5 in total.
- They may be able to work this out in their heads, or they may add the numbers using the column method to get the answer 58.
- The next operation they would need to do is: 58 ÷ 2.
- They should be able to halve 50 in their heads to make 25, and then halve 8 to make 4, then add the 25 and the 4 together to make 29.
I buy a book costing £4.82, a magazine costing £2.97 and a mug costing £3.76. How much money do I spend altogether?
A child would need to work this out very carefully, using a method they were confident with, as this is the kind of calculation where it is easy to make a mistake (confusing pounds with pence, or not lining up digits properly, for example).
I am 1.65 metres tall. My little sister is 93cm tall. How much taller am I than my little sister?
Karen has 1.2 litres of milk. She needs to divide the milk equally into 6 cups. How many millilitres of milk will be in each cup?
It is imperative that children know how to convert between metres and centimetres, litres and millilitres and grams and kilograms before they can tackle these problems. Children need to know how to work out problems like these both using a pencil and paper method and also using a calculator.
Some problems in the SATs are deliberately NOT straightforward! These 'investigation' problems involve children having to think around a problem, possibly by using trial and error, for example:
A sandwich costs 25p more than a biscuit. Louise bought two sandwiches and a biscuit for £2.75. How much does a biscuit cost?
- One way of working this out may be to take a reasonable guess as to how much a sandwich may be.
- Let's imagine the sandwich is 80p, which means the biscuit would be 55p.
- Two sandwiches and a biscuit at these prices would total £2.15.
- Since the total we are trying to get is £2.75, we obviously need to push the prices up a bit.
Try to practise plenty of these kinds of problems or investigations with your child and explain as best you can that trial and error can sometimes be the only 'way in' to a problem!







